What are Imaginary Numbers?
Imaginary numbers are part of the set of complex numbers and are the product of a real number by the imaginary unit i.
Key points
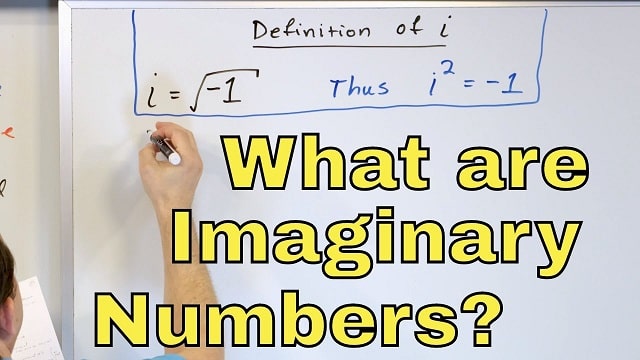
- The imaginary unit i is the square root of -1.
- They allow solving equations with negative square roots and are used in various scientific areas.
- Combined with real numbers, they form complex numbers.
Imaginary Numbers: Simple Explanation
In other words, imaginary numbers are numbers that result from multiplying a real number by the imaginary unit i, allowing equations with negative square roots to be solved and expanding the set of complex numbers.
Imaginary number formula
Given an imaginary number r, this can be expressed as:
r = n·i
where:
- r is an imaginary number.
- n is a real number.
- i is the imaginary unit.
Utility
It may not seem like the idea of imagining numbers is very convincing, but they are actually very useful. Given the previous example, imaginary numbers provide answers to problems that real numbers cannot.
Now when we encounter a negative root we can solve the problem.
Application
Imaginary numbers are widely used in the field of electricity, in quantum mechanics, in Fourier transformations, and combined with real numbers, they create complex numbers, which are also widely used in the field of mathematics.
Curiosity
Imaginary numbers were called imaginary as a mockery because they were conceived as an impossible numerical set and contrary to real numbers.
Imaginary Numbers Example: